This puzzle came up in the New York Times Number Play blog. It goes like this:

An entrepreneur has devised a gambling machine that chooses two independent random variables x and y that are uniformly and independently distributed between 0 and 100. He plans to tell any customer the value of x and to ask him whether y > x or x > y.

If the customer guesses correctly, he is given y dollars. If x = y, he’s given y/2 dollars. And if he’s wrong about which is larger, he’s given nothing.

The entrepreneur plans to charge his customers $40 for the privilege of playing the game. Would you play?

I figured I’d give it a go.  Since I was feeling lazy, and already had my computer in front of me, I thought that I’d do it via simulation rather than working out the exact maths. I tried playing the game with the first strategy that came to mind. If x<50, I would choose y>x, and if x>50, I’d choose yinherent risk aversion of system one. Let’s see how that works out:

N<-100000
x<-sample.int(100,N,replace=TRUE)
y<-sample.int(100,N,replace=TRUE)
dec_rule=50
payout<-numeric(N)
for(i in 1:N)
{
## Correct Guess (playing simple max p(!0) strategy)
if( (x[i]>dec_rule & y[i]<x[i]) | (x[i]<=dec_rule & y[i]>x[i]) )
payout[i]<-y[i]

## Incorrect Guess (playing simple max p(!0) strategy)
if( (x[i]>dec_rule & y[i]>x[i]) | (x[i]<=dec_rule & y[i]<x[i]) )
payout[i]<-0

## Tie pays out y/2
if(x[i] == y[i])
payout[i]<-y[i]/2
}
## Expected Payout ##
print(paste(dec_rule,mean(payout)))

Which leads to an expected payout of $37.75. Playing the risk averse strategy leads to an expected value less than the cost of admission, loosing on average 25 cents per play. No deal, Mr entrepreneur, I had something else in mind for my forty bucks anyway.

Lets try alternate strategies, and see if we can’t play in such a way as to improve our outlook.

## Gambling Machine Puzzle ##
## Puzzle presented in http://wordplay.blogs.nytimes.com/2013/03/04/machine/

result<-numeric(100)
for(dec_rule in 1:100)
{
N<-10000
x<-sample.int(100,N,replace=TRUE)
y<-sample.int(100,N,replace=TRUE)

payout<-numeric(N)

for(i in 1:N)
{
## Correct Guess (playing dec_rule strategy)
if( (x[i]>dec_rule & y[i]<x[i]) | (x[i]<=dec_rule & y[i]>x[i]) )
payout[i]<-y[i]

## Incorrect Guess (playing dec_rule strategy)
if( (x[i]>dec_rule & y[i]>x[i]) | (x[i]<=dec_rule & y[i]<x[i]) )
payout[i]<-0

## Tie pays out y/2
if(x[i] == y[i])
payout[i]<-y[i]/2
}

## Expected Payout ##
print(paste(dec_rule,mean(payout)))
result[dec_rule]<-mean(payout)
}
par(cex=1.5)
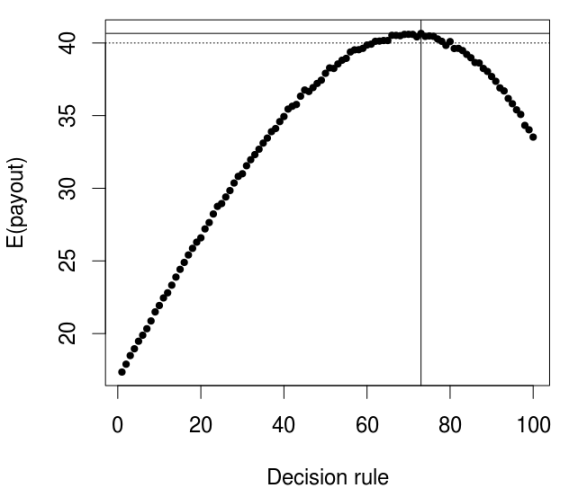
plot(result,xlab='Decision rule',ylab='E(payout)',pch=20)

abline(v=which.max(result))
abline(h=max(result))
abline(h=40,lty=3)

According to which, the best case scenario is an expected payout of $40.66, or an expected net of 66 cents per bet, if you were to play the strategy of choosing y>x for any x<73 and y73. You’re on, Mr entrepreneur!

To calculate the exactly optimal strategy and expected payout, we would need to compute the derivative of the expected payout function with respect to the within game decision threshold. I leave this fun stuff to the reader